Insight into interfacial effect on effective physical properties of fibrous materials. I. The volume fraction of soft interfaces around anisotropic fibers,The Journal of Chemical Physics

当前位置： X-MOL 学术 › J. Chem. Phys. › 论文详情

Our official English website, www.x-mol.net, welcomes your feedback! (Note: you will need to create a separate account there.)

Insight into interfacial effect on effective physical properties of fibrous materials. I. The volume fraction of soft interfaces around anisotropic fibers
The Journal of Chemical Physics ( IF 3.1 ) Pub Date : 2016-01-06 15:20:36 , DOI: 10.1063/1.4939126
Wenxiang Xu _{1,

2,

3} , Han Wang ₁ , Yanze Niu ₁ , Jingtao Bai ₄

Affiliation

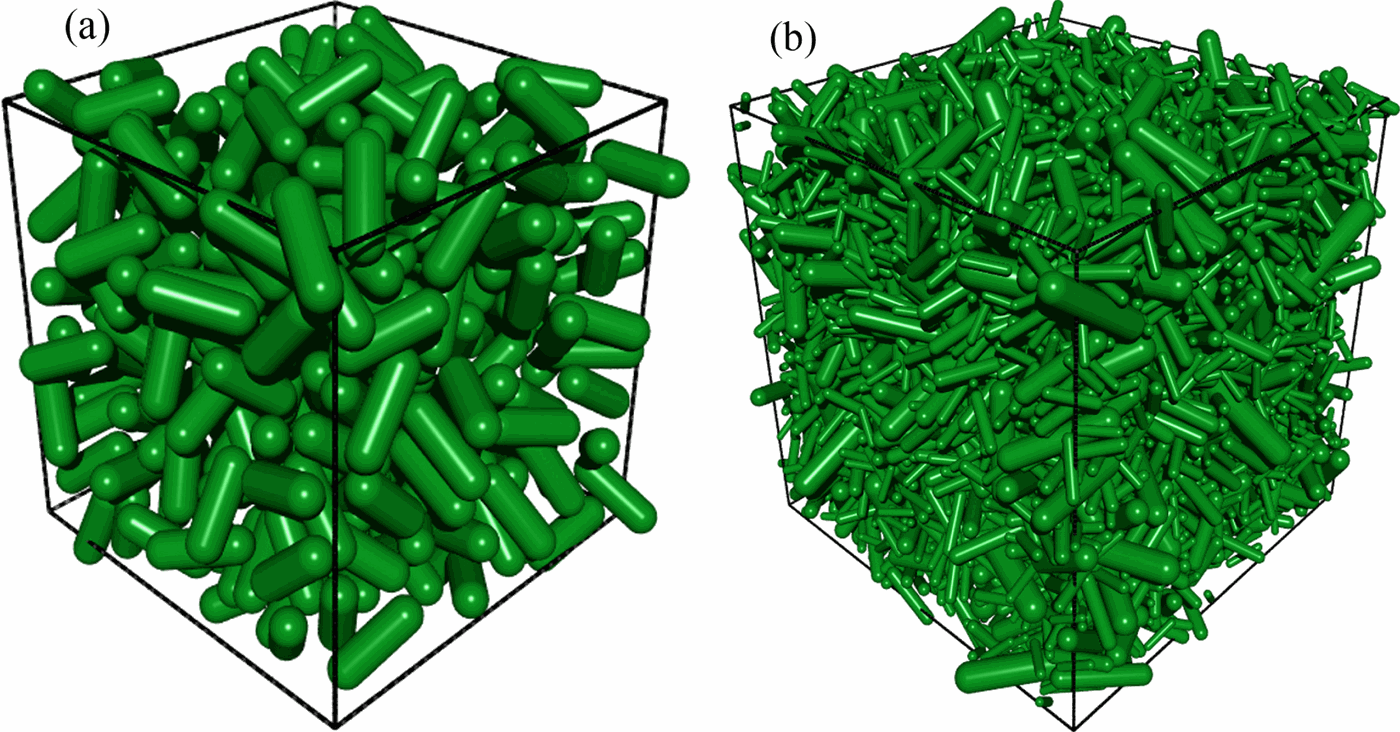
With advances in interfacial properties characterization technologies, the interfacial volume fraction is a feasible parameter for evaluating effective physical properties of materials. However, there is a need to determine the interfacial volume fraction around anisotropic fibers and a need to assess the influence of such the interfacial property on effective properties of fibrous materials. Either ways, the accurate prediction of interfacial volume fraction is required. Towards this end, we put forward both theoretical and numerical schemes to determine the interfacial volume fraction in fibrous materials, which are considered as a three-phase compositestructure consisting of matrix, anisotropic hard spherocylinder fibers, and soft interfacial layers with a constant dimension coated on the surface of each fiber. The interfacial volume fraction actually represents the fraction of space not occupied by all hard fibers and matrix. The theoretical scheme that adopts statistical geometry and stereological theories is essentially an analytic continuation from spherical inclusions. By simulating such three-phase chopped fibrous materials, we numerically derive the interfacial volume fraction. The theoretical and numerical schemes provide a quantitative insight that the interfacial volume fraction depends strongly on the fiber geometries like fiber shape, geometric size factor, and fiber size distribution. As a critical interfacial property, the present contribution can be further drawn into assessing effective physical properties of fibrous materials, which will be demonstrated in another paper (Part II) of this series.

中文翻译：

深入了解界面对纤维材料有效物理性能的影响。一，各向异性纤维周围软界面的体积分数

随着界面性质表征技术的进步，界面体积分数是评估材料有效物理性质的可行参数。然而，需要确定各向异性纤维周围的界面体积分数，并且需要评估这种界面性质对纤维材料的有效性质的影响。无论哪种方式，都需要对界面体积分数的准确预测。为此，我们提出了理论和数值方案来确定纤维材料中的界面体积分数，纤维材料被认为是由基质，各向异性的硬球形圆柱纤维和在其上涂覆有恒定尺寸的软界面层组成的三相复合结构。每根纤维的表面。界面体积分数实际上代表未被所有硬纤维和基质占据的空间的分数。采用统计几何学和立体学理论的理论方案本质上是球形夹杂物的解析延续。通过模拟这种三相切碎的纤维材料，我们从数值上得出了界面体积分数。理论和数值方案提供了定量的见解，即界面体积分数在很大程度上取决于纤维的几何形状，例如纤维形状，几何尺寸因子和纤维尺寸分布。作为一种重要的界面性质，可以将本贡献进一步用于评估纤维材料的有效物理性质，这将在本系列的另一篇论文（第二部分）中得到证明。

更新日期：2016-01-07

点击分享查看原文

点击收藏

阅读更多本刊新发论文