当前位置:
X-MOL 学术
›
J. Chem. Eng. Data
›
论文详情
Our official English website, www.x-mol.net, welcomes your
feedback! (Note: you will need to create a separate account there.)
Solubility Modeling and Mixing Thermodynamics of Thiamphenicol in Water and Twelve Neat Organic Solvents from T = (278.15 to 318.15) K
Journal of Chemical & Engineering Data ( IF 2.0 ) Pub Date : 2017-09-25 00:00:00 , DOI: 10.1021/acs.jced.7b00542 Xinbao Li 1 , Mengqian Wu 1 , Yang Cong 2 , Cunbin Du 2 , Hongkun Zhao 2
Journal of Chemical & Engineering Data ( IF 2.0 ) Pub Date : 2017-09-25 00:00:00 , DOI: 10.1021/acs.jced.7b00542 Xinbao Li 1 , Mengqian Wu 1 , Yang Cong 2 , Cunbin Du 2 , Hongkun Zhao 2
Affiliation
|
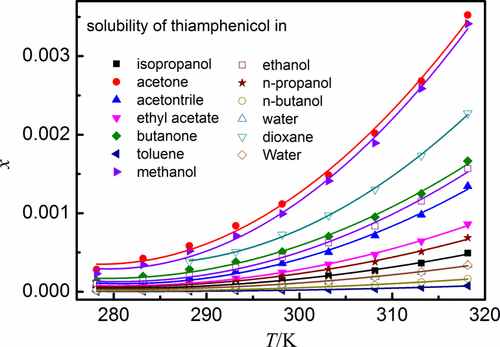
The solid–liquid equilibrium for thiamphenicol in 13 neat solvents (ethanol, methanol, n-propanol, isopropyl alcohol, n-butanol, acetone, acetonitrile, ethyl acetate, 2-butanone, toluene, water, N,N-dimethylformamide, and 1,4-dioxane) was built with the static method at temperatures T = (278.15 to 318.15) K under pressure of 101.2 kPa, and the thiamphenicol solubilities in the selected solvents were measured by using high-performance liquid chromatography. Generally, the solubility data in mole fraction in the selected solvents ranked as N,N-dimethylformamide > acetone > methanol >1,4-dioxane >2-butanone > ethanol > acetonitrile > ethyl acetate > n-propanol > isopropyl alcohol > water > n-butanol > toluene. The nonrandom two-liquid model, Wilson model, modified Apelblat equation, and λh equation were employed to describe the solubility behavior of thiamphenicol in theses solvents. The maximum values of the RMSD and RAD were 4.08 × 10–4 and 2.02%, respectively, and the correlation results by using the modified Apelblat equation were best among the studied models. Additionally, the mixing properties, activity coefficient at infinitesimal concentration and reduced excess enthalpy were derived.
中文翻译:

T =(278.15至318.15)K时,甲砜霉素在水和十二种纯净有机溶剂中的溶解度建模和混合热力学
甲砜霉素在13种纯溶剂(乙醇,甲醇,正丙醇,异丙醇,正丁醇,丙酮,乙腈,乙酸乙酯,2-丁酮,甲苯,水,N,N-二甲基甲酰胺和1)中的固液平衡在静态温度为T =(278.15至318.15)K且压力为101.2 kPa的条件下,通过静态方法制备1,4-二恶烷),并使用高效液相色谱法测定所选溶剂中的噻吩甲酚溶解度。通常,在所选溶剂中以摩尔分数计的溶解度数据为N,N-二甲基甲酰胺>丙酮>甲醇> 1,4-二恶烷> 2-丁酮>乙醇>乙腈>乙酸乙酯>正丙醇>异丙醇>水>正丁醇>甲苯。采用非随机两液模型,Wilson模型,改进的Apelblat方程和λh方程来描述甲砜霉素在这些溶剂中的溶解行为。RMSD和RAD的最大值分别为4.08×10 –4和2.02%,使用改进的Apelblat方程的相关结果在所研究的模型中最好。此外,还得出了混合特性,无限浓度下的活度系数和降低的过量焓。
更新日期:2017-09-25
中文翻译:

T =(278.15至318.15)K时,甲砜霉素在水和十二种纯净有机溶剂中的溶解度建模和混合热力学
甲砜霉素在13种纯溶剂(乙醇,甲醇,正丙醇,异丙醇,正丁醇,丙酮,乙腈,乙酸乙酯,2-丁酮,甲苯,水,N,N-二甲基甲酰胺和1)中的固液平衡在静态温度为T =(278.15至318.15)K且压力为101.2 kPa的条件下,通过静态方法制备1,4-二恶烷),并使用高效液相色谱法测定所选溶剂中的噻吩甲酚溶解度。通常,在所选溶剂中以摩尔分数计的溶解度数据为N,N-二甲基甲酰胺>丙酮>甲醇> 1,4-二恶烷> 2-丁酮>乙醇>乙腈>乙酸乙酯>正丙醇>异丙醇>水>正丁醇>甲苯。采用非随机两液模型,Wilson模型,改进的Apelblat方程和λh方程来描述甲砜霉素在这些溶剂中的溶解行为。RMSD和RAD的最大值分别为4.08×10 –4和2.02%,使用改进的Apelblat方程的相关结果在所研究的模型中最好。此外,还得出了混合特性,无限浓度下的活度系数和降低的过量焓。


















































 京公网安备 11010802027423号
京公网安备 11010802027423号