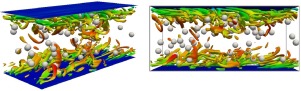
Abstract The lattice Boltzmann method (LBM) is used to simulate turbulent channel flows in the presence of spherical particles. In these simulations, the particles’ surface is fully resolved by relying on the immersed boundary method (IBM). First, a single-phase turbulent flow at a frictional Reynolds number of Re τ = 180 is simulated and used for validation by comparison with published data. The results show very good agreement with reference benchmarks. Starting from these results, a particle-laden flow is considered by direct numerical simulation (LBM-DNS), resolving all relevant scales. Both single-phase and particle-laden flows are modeled at the same frictional Reynolds number by applying the same driving force and initial flow conditions. Particle-to-fluid density ratios of ρ r = 1.0 and 1.2 are considered and the particle radius a is adjusted to either 0.06 or 0.1 times the half-channel height H. The solid phase volume fraction is changed between 0.015 and 0.06. Results of the multiphase cases reveal that the presence of finite-size particles decrease the mean streamwise velocity. In the case of ρ r = 1 and a / H = 0.1 , the mean velocity reduces by 3.0 and 7.9% for volume fractions of 1.5 and 6%, respectively. Attenuation of turbulence by addition of particles is observed as well. The higher the volume fraction, the larger the degree of attenuation. Moreover, particles decrease the maximum streamwise velocity fluctuations by weakening the large-scale streamwise vortices. The root-mean-square of the streamwise velocity component increases in the region very close to the wall and in the core regions. The spanwise and normal velocity fluctuations are increased close to the wall but show minor changes far from the wall. Small particles ( a / H = 0.06 ) cause more reduction of mean streamwise velocity at the same volume fraction in comparison with larger ones. At ϕ = 1.5 % , the maximum rms of streamwise velocity fluctuations with small particles is lower than that with large particles. Finally, heavy particles lead to different velocity profiles in the upper and lower parts of the domain. In all cases, an equilibrium position close to the wall is observed, at which local particle concentration shows a maximum.

中文翻译：

---

球形颗粒存在下湍流通道流动的浸入边界晶格 Boltzmann 模拟

摘要 格子Boltzmann方法(LBM)用于模拟球形颗粒存在下的湍流通道流动。在这些模拟中，依靠浸入边界法 (IBM) 完全解析粒子表面。首先，模拟了摩擦雷诺数 Re τ = 180 的单相湍流，并通过与已发布数据的比较进行验证。结果显示与参考基准非常一致。从这些结果开始，通过直接数值模拟 (LBM-DNS) 考虑载有粒子的流，解析所有相关尺度。通过应用相同的驱动力和初始流动条件，以相同的摩擦雷诺数对单相流和载有颗粒的流进行建模。ρ r = 1.0 和 1 的颗粒与流体密度比。考虑图 2 中的粒子半径 a 调整为半通道高度 H 的 0.06 或 0.1 倍。固相体积分数在 0.015 和 0.06 之间变化。多相情况的结果表明，有限尺寸颗粒的存在降低了平均流向速度。在 ρ r = 1 和 a / H = 0.1 的情况下，对于 1.5% 和 6% 的体积分数，平均速度分别降低 3.0% 和 7.9%。还观察到通过添加粒子对湍流的衰减。体积分数越高，衰减程度越大。此外，粒子通过减弱大规模流向涡流来降低最大流向速度波动。流向速度分量的均方根在非常靠近壁的区域和核心区域中增加。靠近壁面的展向和法向速度波动增加，但远离壁面的变化很小。与较大颗粒相比，小颗粒 (a / H = 0.06) 在相同体积分数下会导致平均流向速度降低更多。在 ϕ = 1.5 % 时，小颗粒的流向速度波动的最大均方根低于大颗粒的。最后，重粒子在域的上部和下部导致不同的速度分布。在所有情况下，观察到靠近壁的平衡位置，在该位置局部粒子浓度显示最大值。5 % 时，小颗粒的流向速度波动的最大均方根低于大颗粒的流向速度波动。最后，重粒子在域的上部和下部导致不同的速度分布。在所有情况下，观察到靠近壁的平衡位置，在该位置局部粒子浓度显示最大值。5 % 时，小颗粒的流向速度波动的最大均方根低于大颗粒的流向速度波动。最后，重粒子在域的上部和下部导致不同的速度分布。在所有情况下，观察到靠近壁的平衡位置，在该位置局部粒子浓度显示最大值。