当前位置:
X-MOL 学术
›
J. Adv. Res.
›
论文详情
Our official English website, www.x-mol.net, welcomes your
feedback! (Note: you will need to create a separate account there.)
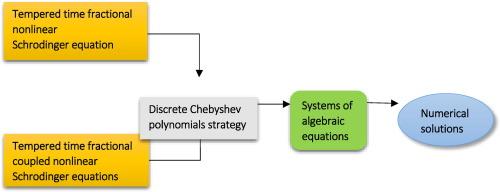
An efficient discrete Chebyshev polynomials strategy for tempered time fractional nonlinear Schrödinger problems
Journal of Advanced Research ( IF 11.4 ) Pub Date : 2024-11-16 , DOI: 10.1016/j.jare.2024.11.014 Mohammad Hossein Heydari, Dumitru Baleanu
Journal of Advanced Research ( IF 11.4 ) Pub Date : 2024-11-16 , DOI: 10.1016/j.jare.2024.11.014 Mohammad Hossein Heydari, Dumitru Baleanu
|
An interesting type of fractional derivatives that has received widespread attention in recent years is the tempered fractional derivatives. These fractional derivatives are a generalization of the well-known fractional derivatives, such as Caputo and Riemann–Liouville. In fact, these derivatives are obtained by multiplying the expressed fractional derivatives by an exponential factor. These fractional derivatives have an additional parameter called λ λ = 0
中文翻译:

一种用于回火时间分数非线性薛定谔问题的高效离散切比雪夫多项式策略
近年来受到广泛关注的一种有趣的分数阶导数是回火分数阶导数。这些分数阶导数是众所周知的分数阶导数(如 Caputo 和 Riemann-Liouville)的泛化。事实上,这些导数是通过将表达的小数导数乘以指数因子获得的。这些分数阶导数有一个称为 λ 的附加参数,因此在 λ=0 的情况下,可以获得经典的 Caputo 或 Riemann-Liouville 分数阶导数。
更新日期:2024-11-16
中文翻译:

一种用于回火时间分数非线性薛定谔问题的高效离散切比雪夫多项式策略
近年来受到广泛关注的一种有趣的分数阶导数是回火分数阶导数。这些分数阶导数是众所周知的分数阶导数(如 Caputo 和 Riemann-Liouville)的泛化。事实上,这些导数是通过将表达的小数导数乘以指数因子获得的。这些分数阶导数有一个称为 λ 的附加参数,因此在 λ=0 的情况下,可以获得经典的 Caputo 或 Riemann-Liouville 分数阶导数。




















































 京公网安备 11010802027423号
京公网安备 11010802027423号