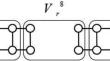
A mixed dominating set in a graph \(G=(V,E)\) is a subset D of vertices and edges of G such that every vertex and edge in \((V\cup E)\setminus D\) is a neighbor of some elements in D. The mixed domination number of G, denoted by \(\gamma _{\textrm{md}}(G)\), is the minimum size among all mixed dominating sets of G. For natural numbers n and k, where \(n > 2k\), a generalized Petersen graph P(n, k) is a graph with vertices \( \{v_0, v_1, \ldots , v_{n-1} \}\cup \{u_0, u_1, \ldots , u_{n-1}\}\) and edges \(\cup _{0 \le i \le n-1} \{v_{i} v_{i+1}, v_iu_i, u_iu_{i+k}\}\) where subscripts are modulo n. In this paper, we explicitly construct an optimal mixed dominating set for generalized Petersen graphs P(n, k) for \(k \in \{1, 2\}\). Moreover, we establish some upper bound on mixed domination number for other generalized Petersen graphs.

图中混合支配集 \（G=（V，E）\） 是 G 的顶点和边的子集 D，使得 \（（V\cup E）\setminus D\） 中的每个顶点和边都是 D 中某些元素的邻居。G 的混合支配数，用 \（\gamma _{\textrm{md}}（G）\） 表示，是所有混合支配 G 集中的最小大小。对于自然数 n 和 k，其中 \（n > 2k\），广义彼得森图 P（n， k） 是一个顶点为 \（ \{v_0， v_1， \ldots ， v_{n-1} \}\cup \{u_0， u_1， \ldots ， u_{n-1}\}\） 和边 \（\cup _{0 \le i \le n-1} \{v_{i} v_{i+1}， v_iu_i， u_iu_{i+k}\}\） 的图，其中下标取模 n。在本文中，我们为 \（k \in \{1， 2\}\） 的广义彼得森图 P（n， k） 显式构建了一个最优混合支配集。此外，我们为其他广义 Petersen 图建立了一些混合支配数的上限。