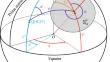
Integral formulas represent a methodological basis for the determination of gravitational fields generated by planetary bodies. In particular, spherical integral transformations are preferred for their symmetrical properties with the integration domain being the entire surface of the sphere. However, global coverage of boundary values is rarely guaranteed. In practical calculations, we therefore split the spherical surface into a near zone and a far zone, for convenience, by a spherical cap. While the gravitational effect in the near zone can be evaluated by numerical integration over available boundary values, the contribution of the far zone has to be precisely quantified by other means. Far-zone effects for the isotropic integral transformations and those depending on the direct azimuth have adequately been discussed. On the other hand, this subject has only marginally been addressed for the spherical integral formulas that are, except for other variables, also functions of the backward azimuth. In this article, we significantly advance the existing geodetic methodology by deriving the far-zone effects for the two classes of spherical integral transformations: (1) the analytical solutions of the horizontal, horizontal–horizontal, and horizontal–horizontal–horizontal BVPs including their generalisations with arbitrary-order vertical derivative of respective boundary conditions and (2) spatial (vertical, horizontal, or mixed) derivatives of these generalised analytical solutions up to the third order. The integral and spectral forms of the far-zone effects are implemented in MATLAB software package, and their consistency is tested in closed-loop simulations. The presented methodology can be employed in upward/downward continuation of potential field observables or for a quantification of error propagation through spherical integral transformations.

积分公式代表了确定行星体产生的引力场的方法基础。特别是，球面积分变换因其对称特性而优选，积分域是球体的整个表面。然而，边界值的全球覆盖很少得到保证。因此，在实际计算中，为了方便起见，我们通过球冠将球面分为近区和远区。虽然近区的重力效应可以通过可用边界值的数值积分来评估，但远区的贡献必须通过其他方式精确量化。各向同性积分变换的远区效应和依赖于直接方位角的远区效应已经得到充分讨论。另一方面，对于球面积分公式，除了其他变量外，球面积分公式也是后向方位角的函数，这一主题仅得到了少量的解决。在本文中，我们通过推导两类球面积分变换的远区效应，显着推进了现有的大地测量方法：（1）水平、水平-水平和水平-水平-水平 BVP 的解析解，包括它们的各个边界条件的任意阶垂直导数的概括以及 (2) 这些广义解析解的空间（垂直、水平或混合）导数高达三阶。远区效应的积分形式和谱形式在MATLAB软件包中实现，并在闭环仿真中测试了它们的一致性。 所提出的方法可用于势场可观测量的向上/向下延拓或用于通过球积分变换来量化误差传播。