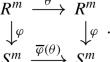
Circulant Column Parity Mixers (CCPMs) are a particular type of linear maps, used as the mixing layer in permutation-based cryptographic primitives like Keccak-f (SHA3) and Xoodoo. Although being successfully applied, not much is known regarding their algebraic properties. They are limited to invertibility of CCPMs, and that the set of invertible CCPMs forms a group. A possible explanation is due to the complexity of describing CCPMs in terms of linear algebra. In this paper, we introduce a new approach to studying CCPMs using module theory from commutative algebra. We show that many interesting algebraic properties can be deduced using this approach, and that known results regarding CCPMs resurface as trivial consequences of module theoretic concepts. We also show how this approach can be used to study the linear layer of Xoodoo, and other linear maps with a similar structure which we call DCD-compositions. Using this approach, we prove that every DCD-composition where the underlying vector space with the same dimension as that of Xoodoo has a low order. This provides a solid mathematical explanation for the low order of the linear layer of Xoodoo, which equals 32. We design a DCD-composition using this module-theoretic approach, but with a higher order using a different dimension.

循环列奇偶校验混合器 （CCPM） 是一种特殊类型的线性映射，用作基于排列的加密原语（如 Keccak-f （SHA3） 和 Xoodoo）中的混合层。尽管应用成功，但对其代数性质知之甚少。它们仅限于 CCPM 的可逆性，并且可逆 CCPM 的集合形成一个组。一种可能的解释是由于用线性代数描述 CCPM 的复杂性。在本文中，我们介绍了一种使用交换代数的模块理论研究 CCPM 的新方法。我们表明，使用这种方法可以推导出许多有趣的代数性质，并且关于 CCPM 的已知结果作为模块论概念的微不足道的结果重新出现。我们还展示了如何使用这种方法来研究 Xoodoo 的线性层，以及其他具有类似结构的线性映射，我们称之为 DCD 组合。使用这种方法，我们证明了与 Xoodoo 具有相同维度的底层向量空间的每个 DCD 组合都具有低阶。这为 Xoodoo 线性层的低阶（等于 32）提供了可靠的数学解释。我们使用这种模块论方法设计了一个 DCD 组合，但使用不同的维度以更高的顺序。