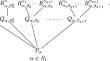
In this work we investigate the problem of producing iso-dual algebraic geometry (AG) codes over a finite field \(\mathbb {F}_{q}\) with q elements. Given a finite separable extension \(\mathcal {M}/\mathcal {F}\) of function fields and an iso-dual AG-code \(\mathcal {C}\) defined over \(\mathcal {F}\), we provide a general method to lift the code \(\mathcal {C}\) to another iso-dual AG-code \(\tilde{\mathcal {C}}\) defined over \(\mathcal {M}\) under some assumptions on the parity of the involved different exponents. We apply this method to lift iso-dual AG-codes over the rational function field to elementary abelian p-extensions, like the maximal function fields defined by the Hermitian, Suzuki, and one covered by the GGS function field. We also obtain long binary and ternary iso-dual AG-codes defined over cyclotomic extensions.

在这项工作中，我们研究了在具有q 个元素的有限域\(\mathbb {F}_{q}\)上生成等对偶代数几何 (AG) 代码的问题。给定函数域的有限可分离扩展\(\mathcal {M}/\mathcal {F}\)和定义在\ (\mathcal {F}\)上的等对偶 AG 代码\(\mathcal {C}\) )，我们提供了一种通用方法，将代码\(\mathcal {C}\)提升为在\(\mathcal {M}上定义的另一个等对偶 AG 代码\(\tilde{\mathcal {C}}\) \)在有关不同指数奇偶性的一些假设下。我们应用这种方法将有理函数域上的等对偶 AG 代码提升为初等阿贝尔p扩展，例如 Hermitian、Suzuki 定义的最大函数域以及GGS函数域所涵盖的最大函数域。我们还获得了通过分圆扩展定义的长二进制和三元异对偶 AG 代码。