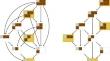
Let L be a finite lattice. Inspired by Ungar’s solution to the famous slopes problem, we define an Ungar move to be an operation that sends an element \(x\in L\) to the meet of \(\{x\}\cup T\), where T is a subset of the set of elements covered by x. We introduce the following Ungar game. Starting at the top element of L, two players—Atniss and Eeta—take turns making nontrivial Ungar moves; the first player who cannot do so loses the game. Atniss plays first. We say L is an Atniss win (respectively, Eeta win) if Atniss (respectively, Eeta) has a winning strategy in the Ungar game on L. We first prove that the number of principal order ideals in the weak order on \(S_n\) that are Eeta wins is \(O(0.95586^nn!)\). We then consider a broad class of intervals in Young’s lattice that includes all principal order ideals, and we characterize the Eeta wins in this class; we deduce precise enumerative results concerning order ideals in rectangles and type-A root posets. We also characterize and enumerate principal order ideals in Tamari lattices that are Eeta wins. Finally, we conclude with some open problems and a short discussion of the computational complexity of Ungar games.

令L为有限格。受 Ungar 对著名斜率问题的解决方案的启发，我们将Ungar 移动定义为将元素\(x\in L\)发送到\(\{x\}\cup T\)的交点的操作，其中T是x覆盖的元素集的子集。下面我们就来介绍一下Ungar游戏。从L的顶部元素开始，两名玩家——Atniss 和 Eeta——轮流做出重要的 Ungar 动作；第一个不能这样做的玩家输掉了游戏。阿特尼斯先上场。如果 Atniss（分别为Eeta）在L上的 Ungar 游戏中有获胜策略，我们就说L是Atniss 胜利（分别为Eeta 胜利） 。我们首先证明Eeta 获胜的\(S_n\)上弱阶主阶理想的数量为\(O(0.95586^nn!)\)。然后，我们考虑杨格中包含所有主阶理想的广泛区间，并描述此类中的 Eeta 胜；我们推导出关于矩形和A型根偏序集的阶理想的精确枚举结果。我们还描述并列举了 Tamari 格子中 Eeta 获胜的主序理想。最后，我们以一些悬而未决的问题和对 Ungar 游戏的计算复杂性的简短讨论作为结束。