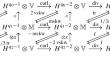
We investigate some Bernstein–Gelfand–Gelfand complexes consisting of Sobolev spaces on bounded Lipschitz domains in \({\mathbb {R}}^{n}\). In particular, we compute the cohomology of the conformal deformation complex and the conformal Hessian complex in the Sobolev setting. The machinery does not require algebraic injectivity/surjectivity conditions between the input spaces, and allows multiple input complexes. As applications, we establish a conformal Korn inequality in two space dimensions with the Cauchy–Riemann operator and an additional third-order operator with a background in Möbius geometry. We show that the linear Cosserat elasticity model is a Hodge–Laplacian problem of a twisted de Rham complex. From this cohomological perspective, we propose potential generalizations of continuum models with microstructures.

我们研究了\({\mathbb {R}}^{n}\)中有界 Lipschitz 域上由 Sobolev 空间组成的一些 Bernstein-Gelfand-Gelfand 复合体。特别是，我们计算了 Sobolev 设置中的共形变形复形和共形 Hessian 复形的上同调。该机制不需要输入空间之间的代数单射性/满射性条件，并且允许多个输入复合体。作为应用，我们使用柯西-黎曼算子和具有莫比乌斯几何背景的附加三阶算子在二维空间中建立了共形科恩不等式。我们证明线性 Cosserat 弹性模型是扭曲 de Rham 复形的 Hodge-Laplacian 问题。从这个上同调的角度来看，我们提出了具有微观结构的连续介质模型的潜在推广。