<br>临界胶束浓度的精确定义及其在膜蛋白研究中使用的烷基麦芽糖苷的应用,RSC Advances

当前位置： X-MOL 学术 › RSC Adv. › 论文详情

Our official English website, www.x-mol.net, welcomes your feedback! (Note: you will need to create a separate account there.)

临界胶束浓度的精确定义及其在膜蛋白研究中使用的烷基麦芽糖苷的应用
RSC Advances ( IF 3.9 ) Pub Date : 2023-03-22 , DOI: 10.1039/d2ra07440k
Adrian Bothe ₁ , Athina Zouni ₁ , Frank Müh ₂

Affiliation

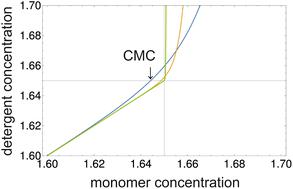
非离子洗涤剂的临界胶束浓度 (CMC) 定义为单体浓度与总洗涤剂浓度的函数关系的临界点，通过将该函数的三阶导数设置为零来确定。结合胶束形成的质量作用模型，该定义给出了 CMC 处单体与总洗涤剂浓度比的解析公式以及 CMC 与胶束化自由能g _mic之间的关系。如果使用 8-苯胺基-1-萘磺酸 (ANS) 或类似探针染料的荧光增强来监测胶束形成，则理论断裂点与实验滴定曲线的断裂点一致。应用到一系列烷基链碳原子数为 8 至 12 的正烷基-β- D-麦芽糖苷，证明了分子热力学模型的良好性能，其中胶束化的自由能由g给出_mic = σΦ + g _pack + g _st 。在该模型中， σ是表面张力量纲的拟合参数， Φ表示与水相接触的疏水分子表面面积的变化， g _pack和g _st分别是烷基链堆积的贡献。胶束内部和洗涤剂头部基团的空间排斥。对不同来源的实验数据的分析表明，如果共溶质与洗涤剂的结合程度不高，则可以通过仅调整σ来解释不同的实验条件（例如水相中的共溶质）。该模型被认为是理论与实用性之间的良好折衷，可应用于膜蛋白的体外研究。

"点击查看英文标题和摘要"

Refined definition of the critical micelle concentration and application to alkyl maltosides used in membrane protein research

The critical micelle concentration (CMC) of nonionic detergents is defined as the breaking point in the monomer concentration as a function of the total detergent concentration, identified by setting the third derivate of this function to zero. Combined with a mass action model for micelle formation, this definition yields analytic formulae for the concentration ratio of monomers to total detergent at the CMC and the relationship between the CMC and the free energy of micellization g_mic. The theoretical breaking point is shown to coincide with the breaking point of the experimental titration curve, if the fluorescence enhancement of 8-anilino-1-naphthalene-sulfonic acid (ANS) or a similar probe dye is used to monitor micelle formation. Application to a series of n-alkyl-β-D-maltosides with the number of carbon atoms in the alkyl chain ranging from 8 to 12 demonstrates the good performance of a molecular thermodynamic model, in which the free energy of micellization is given by g_mic = σΦ + g_pack + g_st. In this model, σ is a fit parameter with the dimension of surface tension, Φ represents the change in area of hydrophobic molecular surfaces in contact with the aqueous phase, and g_pack and g_st are contributions, respectively, from alkyl chain packing in the micelle interior and steric repulsion of detergent head groups. The analysis of experimental data from different sources shows that varying experimental conditions such as co-solutes in the aqueous phase can be accounted for by adapting only σ, if the co-solutes do not bind to the detergent to an appreciable extent. The model is considered a good compromise between theory and practicability to be applied in the context of in vitro investigations of membrane proteins.

更新日期：2023-03-22

点击分享查看原文

点击收藏

公开下载

阅读更多本刊新发论文本刊介绍/投稿指南