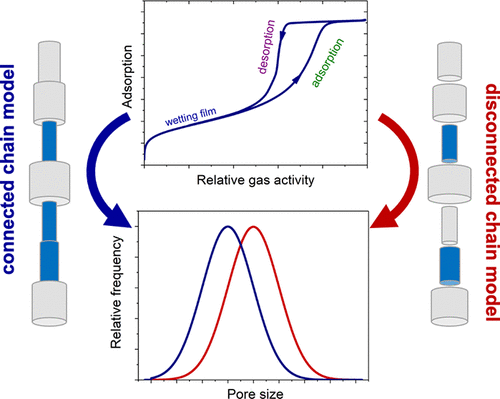
Valiullin et al. [J. Phys. Chem. C2019, 123, 16239] have developed a statistical theory describing adsorption/desorption hysteresis in one-dimensional pore structures with structural disorder. The theory captures the behavior of such systems based on simple rules for the phase transformations in individual pores together with assumptions about the role of pore connectivity. To test this theory, we have developed an algorithmic method for exact construction of sorption isotherms for chains of pores from single pore isotherms. We show that the predictions of the statistical theory are indeed very accurate. We have used the statistical theory to extract the pore size distribution (PSD) and chain lengths using both adsorption and desorption branches of the isotherm obtained by the algorithmic model on various pore structures. The predicted PSD’s and chain lengths are in excellent agreement with the actual properties of the original structures. We conclude that the statistical theory offers a new direction toward a more accurate characterization methodology of nanoporous materials.

中文翻译：

---

使用算法和统计模型的串联孔隙模型（SCPM）结构的吸附等温线重建和孔隙大小分布的提取

Valiullin等。[ J. Phys。化学 Ç 2019，123[16，16239]已经发展了一种统计理论，描述了具有结构紊乱的一维孔结构中的吸附/解吸滞后。该理论基于有关单个孔中相变的简单规则以及关于孔连通性作用的假设，来捕获此类系统的行为。为了验证该理论，我们开发了一种算法方法，可精确构造单个孔等温线中孔链的吸附等温线。我们证明了统计理论的预测确实非常准确。我们已经使用统计理论来提取通过等温线的吸附和解吸支链通过算法模型在各种孔结构上获得的孔径分布（PSD）和链长。预测的PSD和链长与原始结构的实际特性非常吻合。我们得出结论，统计理论为纳米孔材料更精确的表征方法提供了新的方向。